First Order Frequency Response

Frequency response is is the response of a system to a sinusoidal/oscillating input.

Response to Sinusoidal input

For a standard first order system $T\frac{d}{dt}y(t)+y(t)=u(t)$, with a sinusoidal input $u(t)=Asin(\omega t)$:

$$U(s)=\mathcal{L}(u(t))=\frac{A\omega }{s^2+{\omega }^2}$$ $$Y(s)=U(s)G(s)=\frac{A\omega }{s^2+{\omega }^2}\frac{1}{Ts+1}$$ $$y(t)={\mathcal{L}}^{-1}(Y(s))=\frac{A}{\sqrt{1+{\omega }^2{T}^2}}sin(\omega t-{\tan }^{-1}\omega T)+\frac{A\omega T}{1+{\omega }^2{T}^2}{e}^{-\frac{t}{T}}$$

The sinusoidal part of the equation is the steady-state that the response tends to, and the exponential part is the transient part that represents the rate of decay of the offset of the oscillation.

• The frequency of input and output is always the same
  • It is the amplitude and phase shift $\varphi$ that change
  • These depend on the input frequency $\omega$
    • This dependence is the frequency response

Example

The example below shows an input $sin(4\pi t)$, and its output with $G(s)=\frac{1}{s+1}$

$$y(t)=0.0793sin(4\pi t+\varphi )+0.079e^{-t},\varphi =-{\tan }^{-1}4\pi =-85^{\circ }$$

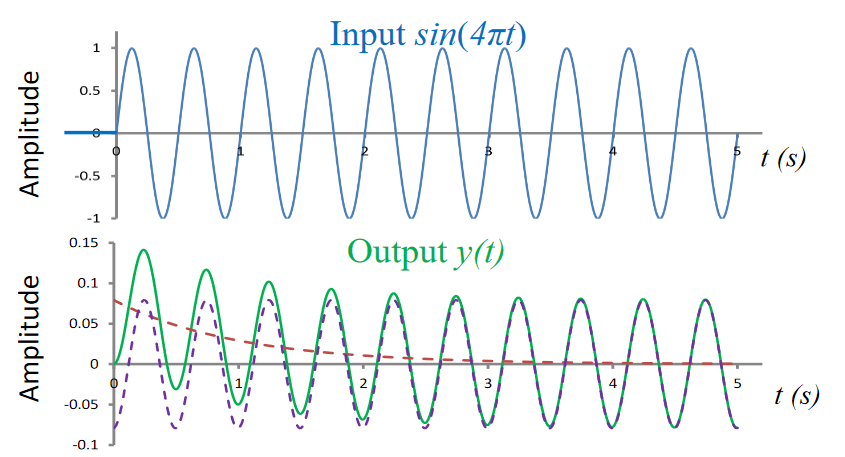
The steady state sinusoidal and transient exponential part of this response can be seen in the equation.

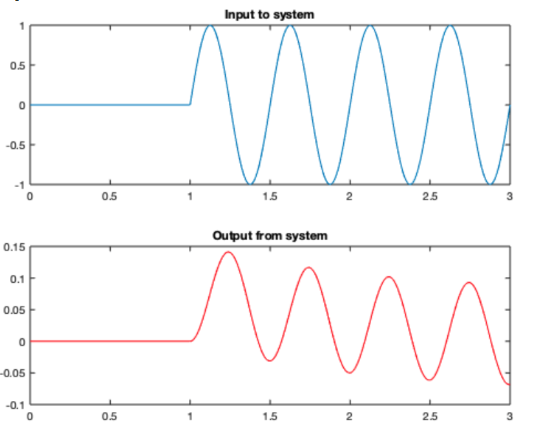
Matlab Example

The following code generates the following plot

system = tf(1,[1 1]);
t = 0:0.01:3; % time value vector
u = (t>=1).*sin(4 * pi * t) %input signal for t >= 1
y = lsim(sys,u,t); % simulate system with input u

figure;
subplot(2,1,1); plot(t,u); title("input");
subplot(2,1,2); plot(t,y,'r'); title("outputA");

Gain and Phase

Gain is the ratio of output to input amplitude, ie how much bigger or smaller the output is compared to input.

$$G=\frac{E}{A}(\omega )$$

Phase difference $\varphi (\omega )$ is how much the output signal is delayed compared to the input signal. Both are functions of input frequency $\omega$.

The frequency response can be obtained by substituting $j\omega$ for $s$ in the transfer function. This gives a complex function as shown

$$G(s)=\frac{1}{Ts+1}⟹G(j\omega )=\frac{1}{Gj\omega +1}$$

Magnitude $|G(j\omega )|$ gives the amplitude of the response, and the argument of the complex number $\angle G(j\omega )$ gives the phase shift $\varphi$. The substitution $s=j\omega$ is used, is because in the Laplace domain, both signals and systems are represented by functions of $s$.

• The $s$-plane is the complex plane on which Laplace transforms are graphed.
• Generally, $s=\sigma +j\omega$
• $\sigma$ is the Neper frequency, the rate at which the function decays
• $\omega$ is the radial frequency, the rate at which the function oscillates
• Periodic sinusoidal inputs are non decaying, so $\sigma =0$, giving $s=j\omega$

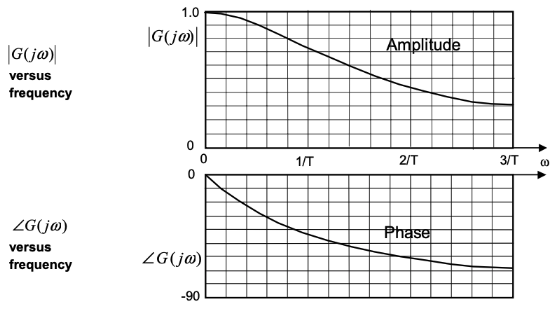
To find the frequency response parameters:

$$G(j\omega )=\frac{1}{1+j\omega T}\times \frac{1-j\omega T}{1-j\omega T}=\frac{1-j\omega T}{1+{\omega }^2{T}^2}$$ $$=\frac{1}{1+{\omega }^2{T}^2}-j\frac{\omega T}{1+{\omega }^2{T}^2}$$ $$=Re(G)-jIm(G)$$ $$|G(j\omega )|=\sqrt{(Re(G){)}^2+(Im(G){)}^2}=\frac{1}{\sqrt{1+{\omega }^2{T}^2}}$$

$$\angle G(j\omega )={\tan }^{-1}\frac{Im(G)}{Re(G)}=-{\tan }^{-1}\omega T$$

The graphs below show the frequency response in terms of $T$ for varying frequency $\omega$:

Example

Given a transfer function $G=\frac{1}{s}$, what is the magnitude and phase of frequency response? $$G(j\omega )=\frac{1}{j\omega }=\frac{-j}{\omega }=0-\frac{1}{\omega }j$$ $$|G(j\omega )|=\sqrt{\frac{1}{{\omega }^2}}=\frac{1}{\omega }$$ $$\angle G(j\omega )={\tan }^{-1}\frac{\frac{-1}{\omega }}{0}=-\frac{\pi }{2}$$

Bode Plots

Bode plots show frequency and amplitude of frequency response on a log${}_{10}$ scale. Information is not spread linearly accross the frequency range, so it makes more sense to use a logarithmic scale. An important feature of bode plots is the corner frequency: the frequency at the point where the two asymptotes of the magnitude-frequency graph. This point is where $\omega =\frac{1}{T}$.

The plot above is for the function $G(s)=\frac{1}{s+1}$. The gain is measured in decibels $dB$ for the magnitude of the response.