Narrowband Matching

A transmission line of characteristic impedance $Z_0$ is matched to a load $Z_L$ when $Z_L=Z_0$: not incident waves upon the load are reflected back at the source. A matching network is used to achieve these conditions, placed between the load and the line. Examples of matching networks include

• The $\lambda /4$ transformer
  • A transmission line in series of length $\lambda /4$
• A capacitor/inductor in shunt
• A short circuit stub in parallel

Note that for lines of length $l=n\lambda /2$, since $\beta l=n\pi$, the input impedance of the line is equal to the load impedance and the line does not modify the impedance of the load to which it is connected.

$$Z_{in}=Z(z=-l)=Z_0\frac{Z_L+j{Z}_0\tan (n\pi )}{Z_0+j{Z}_L\tan (n\pi )}=Z_L$$

$\lambda /4$ Transformer

The input impedance of a line of length $\lambda /4$ is

$$Z_{01}=Z_{in}=Z_{02}\frac{Z_L+j{Z}_{02}\tan (\pi /2)}{Z_{02}+j{Z}_L\tan (\pi /2)}=\frac{Z_{02}^2}{Z_L}$$

$$Z_{02}=\sqrt{Z_{01}{Z}_L}$$

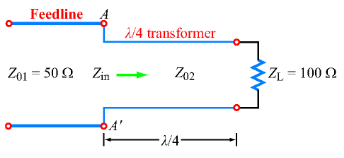
This eliminates reflections at $A{A}^{\prime }$ to make $Z_{01}=Z_{in}$.

\Gamma = \frac{Z_{in} - Z_0}{Z_{in_} + Z_0}

At the frequency for which the transformer is a perfect $\lambda /4$, there is a perfect match, and $\Gamma =0$. However, as we deviate from the match frequency, the performance degrades:

$$|\Gamma |=\frac{1}{(1+(4Z_0{Z}_L/(Z_L-Z_0{)}^2){\sec }^2\beta \lambda {)}^{1/2}}$$

We use ${\Gamma }_m$ as an acceptable maximum reflection coefficent, for which the bandwith is defined:

$$\Delta \theta =2\left(\frac{\pi }{2}-{\theta }_m\right)$$

Solving for $\cos {\theta }_m$ from the above equations gives:

$$\cos {\theta }_m=\frac{{\Gamma }_m}{\sqrt{1-{\Gamma }_m^2}}\frac{2\sqrt{Z_0{Z}_L}}{|Z_L-Z_0|}$$

Assuming TEM lines, where $f_0$ is the designed frequency, we can then link ${\theta }_m$ with $f_m$, the max/min frequency at which our match has an acceptable performance:

$$\theta =\beta l=\frac{2\pi f}{u_p}\frac{u_p}{4f_0}=\frac{\pi f_m}{2f_0}{\theta }_m=\frac{\pi f_m}{2f_0}$$

The fractional bandwith of a matching section (where $\cos {\theta }_m$ is derived above):

$$\frac{\Delta f}{f_0}=\frac{2(f_0-f_m)}{f_0}=2-\frac{2f_m}{f_0}=2-\frac{4{\theta }_m}{\pi }$$

The smaller the load mismatch, the larger the bandwidth.

Lumped Element Matching Networks

An L-secion uses two reactive elements to match a load impedance to a transmission line. If $Z_L$ falls within the $1+jx$ circle on the Smith chart, then the left configuration is used, else the right configuration is used.

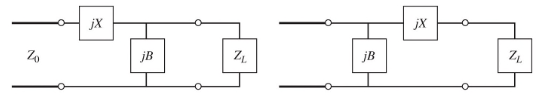
Let $Z_L=R_L+j{X}_L$ where $R_L>Z_0$ (inside $1+jx$ circle). For an impedance match:

$$Z_0=jX+{\left(jB+\frac{1}{R_L+j{X}_L}\right)}^{-1}$$

Solving for $X$ and $B$:

$$B=\frac{X_L\pm \sqrt{R_L/Z_0}\sqrt{R_L^2+X_L^2-Z_0{R}_L}}{R_L^2+X_L^2}$$

$$X=\frac{1}{B}+\frac{X_L{Z}_0}{R_L}-\frac{Z_0}{B{R}_L}$$

Two solutions are possible, and both are physically realisable with capacitors/inductors.

Conside the alternative where $R_L<Z_0$ (outside $1+jx$ circle):

$$\frac{1}{Z_0}=jB+\frac{1}{R_L+j(X+X_L)}$$

$$X=\pm \sqrt{R_L(Z_0-R_L)}-X_{L}B=\pm \frac{\sqrt{(Z_0-R_L)/R_L}}{Z_0}$$

Shunt Lumped Element Matching

We use a lumped element in parallel with the load to achieve matching as shown in the figure. As the element $Y_s$ is in shunt, we work in the admittance domain.

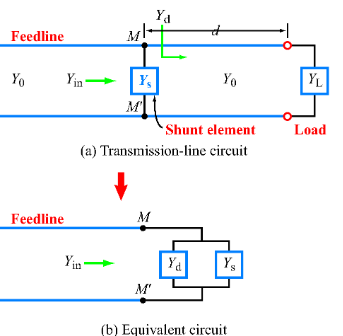
Assuming $Z_L=R_L+j{X}_L$, the aim is at terminal $M{M}^{\prime }$ to transform $R_L$ to $Z_0$, and $X_L$ to $0$. Assuming $Y_s=j{B}_s$ and $Y_d=G_d+j{B}_d$, the aim is to choose a length $d$ and value of $Y_s$ to match $Y_0$ of the feedline to $Y_{in}$, given by the sum of $Y_d$ and $Y_s$.

$$Y_{in}=j{B}_s+G_d+j{B}_s=G_d+j(B_s+B_d)$$

$$G_d=Y_0=1/Z_0{B}_s+B_d=0$$